One of the fourth grade teachers I work with had a fantastic idea for reviewing measurement. She said she was frustrated tripping over gumballs from the tree outside the school and it could be really fun to review measurement (the concept her students had been working on) using this problem-base d situation as a springboard. She asked if I could help her come up with some activities for her lesson the following day and she would work on some too.
d situation as a springboard. She asked if I could help her come up with some activities for her lesson the following day and she would work on some too.
I put together some activities for her using the Gumball Character for the TV show The Amazing World of Gumball as the theme while I was participating in a Twitter chat (her version turned out way better!). While I was working on my version, I decided this would also be a great way to review multiplication using arrays and would create a great entry point for all students.
She set up the activity by telling students they would all start using the multiplication sheet by making arrays and then writing down arrays others had made. They didn’t have to draw the array (there was space if they wanted to), but they did have to write the corresponding multiplication equation. After a few safety tips and discussion about determining which tools they would need for the measurement portion, we headed outside.
Arrays
We had several surprises as students completed the array activity. Some students were just counting gumballs and then trying to figure out the multiplication equation that would go with it, which was fine until they got to a prime number. We prompted with,
“Make an array using those gumballs.”
Some needed a review as to what an array looked like, once that was cleared up they were on their way.
Other students were making arrays, but not sure how to set up the equation when they encountered factors they did not have memorized (red flag!) such as 14 x 2 or 7 x 6. We asked things like,
“How could we partition the array into friendlier facts?”
For some students that was enough, for others we had to partition it and then follow up with
“Do you see 10 groups of 2? How many groups of 2 are left?”
Extensions
This got me to thinking about extensions to the activity such as:
Get a few handfuls of gumballs. Write the factors and the product
or Write the corresponding division sentence. How can we represent the situation if it is a prime number?
This would be a great introduction or review of prime numbers and lead to a discussion of order of operations such as 19 = 2 x 9 +1 or division with remainders, which really opens up this activity to fifth grade students as well.
In third grade, this could be used as students explore arrays and strategies for multiplication and division. Students could write the fact families for each array or the strategies they used to solve the multiplication sentences. Also great as they are learning about the commutative property.
Measurement
As students began their measurement activities, we noticed even more misconceptions. One of the problems that got misinterpreted was “The number of gumballs that fell in one square meter.” Some students started measuring the perimeter of one square meter and others tried to find a square (sidewalk) that measured one square meter. Many groups required prompting for this such as,
“Let’s reread the question. It says…” “Where did the gumballs fall?”
Students: “over there!”
“So where should we be measuring?”
Once they realized that they would be finding the gumballs that fell, many students still did not know where to start. We asked,
“What would one square meter look like?” “How could we measure that?
We only had one meter stick so students were having a hard time figuring out how to make the square. We asked,
“How many centimeters in a meter?” “Do we have a tool that would measure that?” “How could that help you?”
This was really the prompt that led to what you see in the pictures. Students started to realize that they could lay measuring tapes on each side. However, when I went to look at their squares, many had not measured to the 100 cm mark on each side, they had used the entire tape. I then prompted with,
“How many centimeters should be on each side?” “Is that what you have here?”
Once that was established, some students thought they would then fill the square meter with gumballs and count it. After all that’s what we do when we practice area in third grade. We count the square units inside. This led us to ask,
“Let’s go back to the question. What does it ask?”
Some still needed more prompting such as,
“So how could we find the number of gumballs in the square meter you just made?”
Students, “Count them?”
“Would that answer the question?”
Students, “Yes.”
“Then it sounds like you have a plan.”
We (but most importantly, the students) really enjoyed this opportunity to get outside and get our hands dirty. The great thing is that while completing these activities, students managed to clean up over 10 paper bags of gumballs from the ground outside our school!
Student engagement was off the charts and the activity allowed for multiple entry points.
One of the students who has an intellectual disability also decided to use a pan balance using rocks and gumballs to explore. It created the opportunity to discuss comparison of numbers and weights.
Here is a link to some of the activities. The document Mrs. Anderson made is more inclusive and I will add that once she sends me the link.
There is also a link to a American Forests site that I think could be a cool extension on measurement of the height of trees.
If you don’t have gumball trees at your school, try using rocks or sticks or other seedpods!














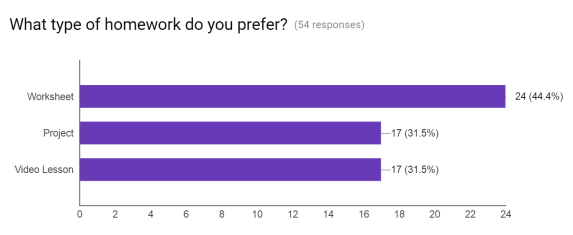

 We decided to open up the school in the morning to them, do a week of project based homework and a week of flipped lessons and give the survey again. We also decided to start with 4th grade math and bring in 5th grade when we could gauge the number of students we would have in attendance. We are sending home a letter to parents explaining the change and will start next week!
We decided to open up the school in the morning to them, do a week of project based homework and a week of flipped lessons and give the survey again. We also decided to start with 4th grade math and bring in 5th grade when we could gauge the number of students we would have in attendance. We are sending home a letter to parents explaining the change and will start next week! students immediately started solving the problem and raising their hands to tell me the solution. I reiterated the fact that I was not looking for a solution, I simply wanted them to think about how we would represent 1 or one whole in fraction form.
students immediately started solving the problem and raising their hands to tell me the solution. I reiterated the fact that I was not looking for a solution, I simply wanted them to think about how we would represent 1 or one whole in fraction form.









 d situation as a springboard. She asked if I could help her come up with some activities for her lesson the following day and she would work on some too.
d situation as a springboard. She asked if I could help her come up with some activities for her lesson the following day and she would work on some too.
















